- The first step of experiment 1 is what is called clock synchronization.
Clock synchronization starts from a common light source, also called a reset signal.
Clock synchronization starts from the idea that the light path from the light source towards each clock should be the same.- In the case of 2 clocks this distance is 0.5*l. The two clocks are #1 and #2. To synchronize, the light source should be in between the 2 clocks, because the distance between clock #1 and clock #2 is each 0.5*l and the same.
- In the case of 4 clocks this distance is 1.5 * l. The four clocks are #1,#2,#3 and #4
The light source is in between points #2 and #3. From there it goes to two new reset points: one reset point in between the points #1 and #2 and one in between the points #3 and #4. Light distance is l.
The first reset point is used to reset the clocks #1 and #2. The second reset point is used to reset the points #3 and #4. Total light distance is l+0.5l = 1.5l. - In the case of 8 clocks this distance is 3.5 * l. The eight clocks are #1 through #8
The light source is in between the points #4 and #5. From there it goes to two new reset points: one reset point in between the points #2 and #3 and one in between the points #6 and #7. Light distance is 2.
The first reset point in between the points #2 and #3 is used to create two new reset points: one between the points #1 and #2 and one between the points #3 and #4
The second reset point in between the points #6 and #7 is used also to create two new reset points: one between the points #5 and #6 and one between the points #7 and #8.
Each of these 4 reset points is used to reset the two nearby clocks. Total light distance = 2l+1l+0.5l = 3.5l
- The second step is what is called: moving clock synchronization
moving clock synchronization comes in two flavours.
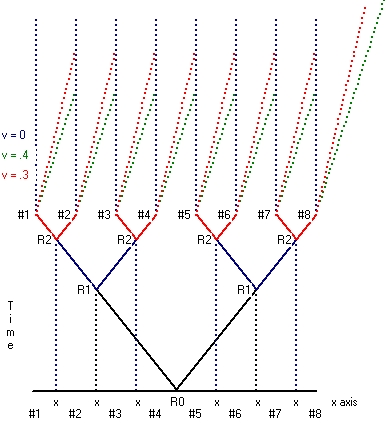
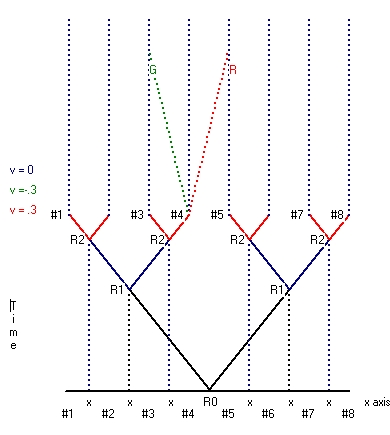
- The first type starts from a situation where the reset point is considered at rest. This type is depicted in Picture 1. Picture 1 shows Clock synchronization for 8 clocks along the x-axis. In the case of moving clock synchronization a rod is used which also contains 8 clocks (example) at the same position as each of the 8 clocks along the x-axis. That means when the reset signal is 16 clocks are synchronized at rest. This is the first part.
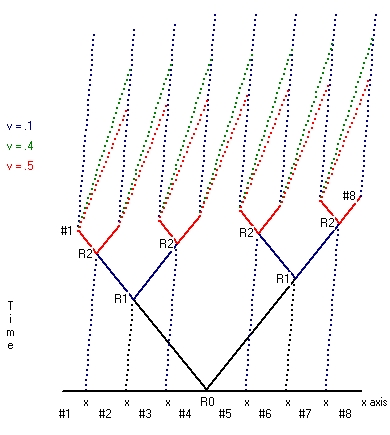
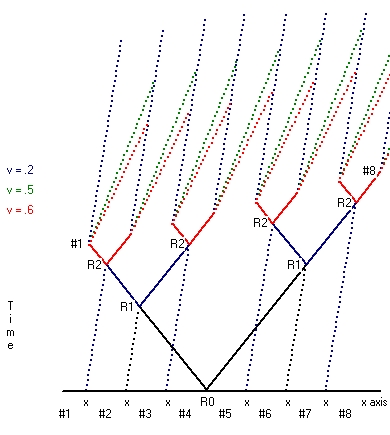
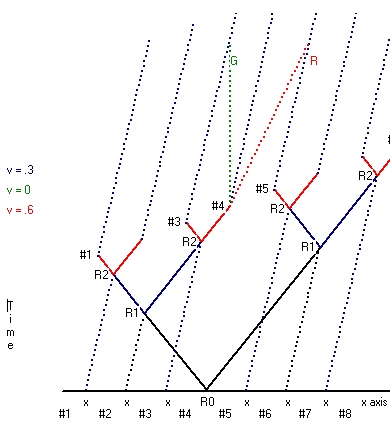
The second part is that the rod is moved. During this movement all the 8 moving clocks stay synchronized, but the clocks all run synchronic slower. When the movement stops all the 16 clocks tick at the same rate, but the 8 clocks moved run behind versus the 8 clocks which stayed at rest. - The second type starts from the situation where the reset point is not considered at rest. This type is shown in Picture 2 for both v=0.2*c and v=0.4*c.
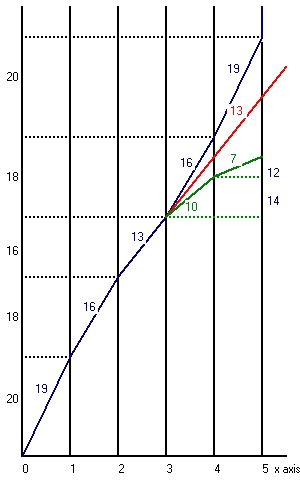
Starting point is exactly the same as in Picture 1. Initially there are 8 clocks equal spaced a fixed distance l apart. There is a reset point in between the clocks #4 and #5. The whole setup is considered to move towards the right. The speed of light is again considered to be the same in all directions.
When this is the case what Picture 2 shows is that clock #1 receives the reset signal physical first, then clock #2, then clock #3 etc. What Picture 2 also shows is that the time difference between clock #2 and clock #1, compared with clock #3 and clock #2 is the same
- The first type starts from a situation where the reset point is considered at rest. This type is depicted in Picture 1. Picture 1 shows Clock synchronization for 8 clocks along the x-axis. In the case of moving clock synchronization a rod is used which also contains 8 clocks (example) at the same position as each of the 8 clocks along the x-axis. That means when the reset signal is 16 clocks are synchronized at rest. This is the first part.
- The third step indicates the beginning of the experiment. This means that the engine that moves the rod is turned on.
At the front end of the rod there is an observer which observes both the readings of the clock at rest and the moving clock when he passes the next clock at rest.
The following table shows the results when the observer in front passes the clocks #3 to #9
The clock readings in counts represent the increments between each reading. To get the actual counts observed you have to add these increments.clock v0 n0 v1 v^2 c^2 gamma n1 time 0 time 1 2 0 0 0 100 100/100 3 0 1000 1 1 100 99/100 990 1000 990 4 0 500 2 4 100 96/100 480 1500 1470 5 0 333 3 9 100 91/100 303 1833 1773 6 0 250 4 16 100 84/100 210 2083 1983 7 0 200 5 25 100 75/100 150 2283 2133 8 0 166 6 36 100 64/100 106 2449 2239 9 0 166 6 36 100 64/100 106 2615 2345 Table 1 - Column 1 shows the clock # for the observer in front.
- Column 2 v0 shows the speed of the rod as measured with the clocks at rest.
- Column 3 n0 shows the # of counts of the clock at rest. The # of counts is an indication of time passed.
What the full table shows is that the number of counts (increments) decreases because the speed in creases. - Column 4 v1 shows the speed of the rod as measured with the clocks at rest. v1 = 1000/t = 1000/n0. The speed of light c = 10.
- Column 5 v^2 shows the speed of the rod v1 in the power of two
- Column 6 c^2 shows the speed of light in the power of two. c^2 = 10^2 = 100
- Column 7 gamma shows the factor gamma. Gamma is defined as (1-v^2/c^2).
- Column 8 n1 shows the # of counts of the moving clock. The # of counts of the moving clock is lower than the clocks at rest, which mean that the moving clock runs physical slower; the moving clock runs behind.
- Column 9 time 0 shows the time (in counts) of the clock at rest. This is the sum of column 2 i.e. n0
- Column 10 time 1 shows the time (in counts) of the moving clock. This is the sum of column 7 i.e. n1
- The fourth step involves the observer at the back of the rod. This observer also observes both the readings of the clock at rest and the moving clock when he passes the next clock at rest.
The interesting part is that he writes down exactly the same results as the observer in front.
The reason is physical because the whole rod undergoes the same physical changes and forces at each instant.
The moving clocks once synchronized, stay synchronized during the whole trip.
However, the moving clocks are not synchronized with the clocks at rest. The moving clocks run slower. - The fifth step involves the physical situation that the rod undergoes accelerations. That means the rods is during part of this trip in non-linear movement. This is the physical reason why the moving clock runs slower as the clock at rest.
Its light path is longer as the clock at rest.
When the position of the observer in front coincides with the clock at rest #8 the engine, which moves the rod, is turned off. - From there on the rod is in linear movement. The same for the observer at the back with clock #8.
- When studying the physical behaviour of the moving rod it is important to notice that it does not matter in which direction the moving rod behaves. This whole experiment is symmetrical.
The next table shows the situation for the same clock as above, but now moving in opposite direction.clock n0 v0 v1 v^2 c^2 gamma n1 time 0 time 1 2 0 0 0 100 100/100 1 1000 0 -1 1 100 99/100 990 1000 990 0 500 0 -2 4 100 96/100 480 1500 1470 -1 333 0 -3 9 100 91/100 303 1833 1773 -2 250 0 -4 16 100 84/100 210 2083 1983 Table 2